Difference Quotient: Definition, Formula, Properties & Solved Examples

The difference quotient is a mathematical formula used to calculate the average rate of change of a function over a given interval, often represented as $\ \dfrac{f(x + h)-f(x)}{h}. \$ It serves as a foundational tool in calculus, leading to the concept of derivatives and helping analyze the behavior of functions. The difference quotient measures the slope of the secant line between two points on a graph.

Get Homework Help

1. Introduction to the Difference Quotient:

In mathematics, especially in calculus, the difference quotient is a critical concept. It forms the foundation of understanding derivatives, slopes of curves, and rates of change. Whether you're a student looking to grasp this idea for the first time or someone revisiting it, the difference quotient offers significant insights. As we walk through this blog, we will dissect its formula, the process of finding it, its properties, and much more.

2. What is the Difference Quotient:

The difference quotient is a mathematical formula used to compute the average rate of change of a function over a specified interval. Essentially, it helps calculate the slope of the secant line between two points on a function's graph. By examining the ratio of the change in the function's output to the change in input, we get a snapshot of how the function behaves over an interval.

In simpler terms, imagine driving a car. The difference quotient is like calculating your average speed between two points. You’re not worried about the instantaneous speed at any specific point (yet), but more about how fast you’re going on average from point A to point B.

3. Difference Quotient Formula:

The difference quotient formula is expressed as follows:

$\dfrac{f(x + h) - f(x)}{h}$

Where:

• $f(x)$ represents the function.

• $h$ is the distance between the two points on the x-axis.

• $f(x + h)$ represents the function evaluated at the point $(x + h)$.

This formula is used to compute the rate of change in the function as $h$ approaches zero. As $h$ gets smaller, the difference quotient gives us a clearer picture of the instantaneous rate of change, ultimately leading us to the derivative.

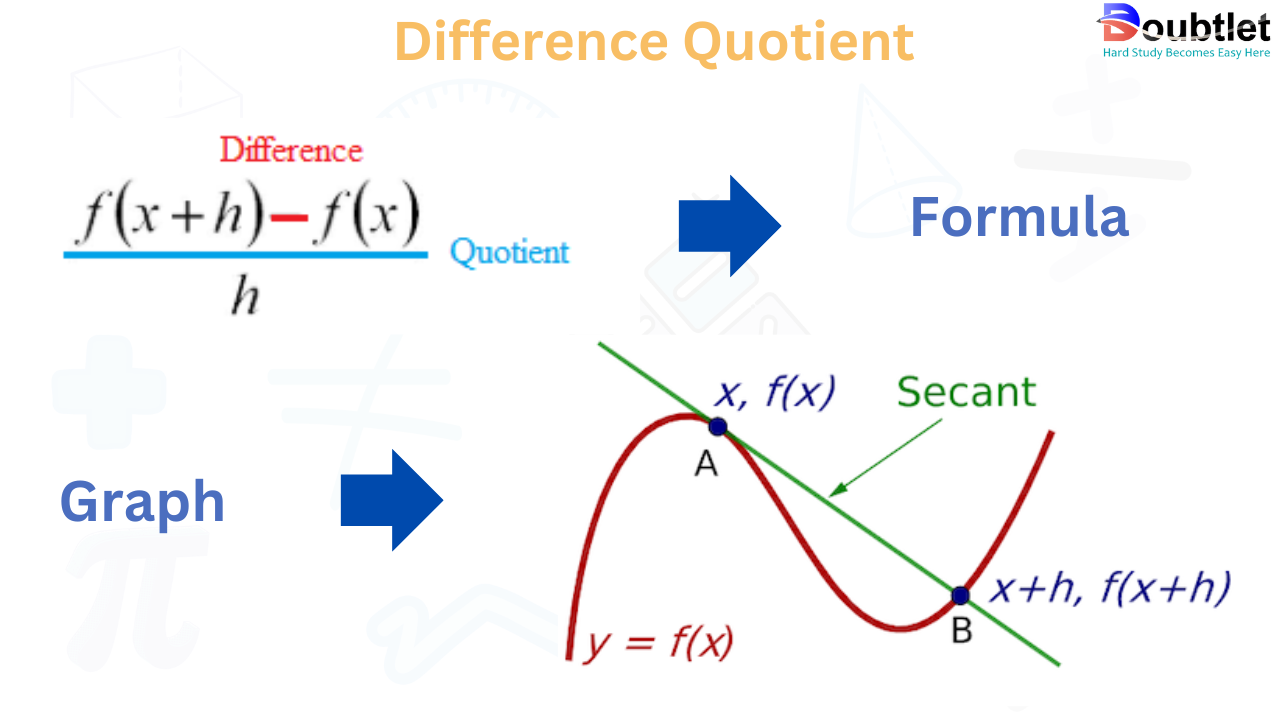
4. How to Find the Difference Quotient:

To find the difference quotient, follow these simple steps:

1. Step 1: Identify the function, $f(x)$.

2. Step 2: Determine the expression $f(x + h)$. This means replacing $x$ with $(x + h)$ in the original function.

3. Step 3: Subtract $f(x)$ from $f(x + h)$.

4. Step 4: Divide the result by $h$.

While it sounds simple, getting each step right ensures accuracy in understanding how the function changes over a small interval. The difference quotient lays the groundwork for calculating derivatives, making it a cornerstone in calculus.

5. Rules for Finding the Difference Quotient:

There are a few fundamental rules you should follow when working with the difference quotient:

• Substitution: When calculating $f(x + h)$, ensure that every instance of $x$ in the original function is correctly substituted with $x + h$. Mistakes in substitution lead to incorrect results.

• Simplification: After performing the subtraction $f(x + h) - f(x)$, it’s crucial to simplify the expression before dividing by $h$.

• Handling $h$: Make sure that $h$ doesn’t equal zero, as division by zero is undefined in mathematics. However, we eventually take the limit of $h$ approaching zero when moving to derivatives.

6. Properties of Difference Quotient:

The difference quotient has certain key properties that are essential to remember:

• Rate of Change: It represents a function's average rate of change over an interval.

• Secant Line Slope: The quotient gives the slope of the secant line between two points on the curve of the function.

• Approximates Derivative: As $h$ approaches zero, the difference quotient approximates the function's derivative at a point.

These properties make it a versatile tool for understanding the behavior of functions and laying the groundwork for advanced calculus concepts.

7. Difference Quotient Solved Examples:

Question: 1

Given the function $f(x) = x^2$, find the difference quotient.

Solution:

• Step 1: Find $f(x + h)$.
  $f(x + h) = (x + h)^2 = x^2 + 2xh + h^2$

• Step 2: Subtract $f(x)$ from $f(x + h)$.
  $(x^2 + 2xh + h^2) - x^2 = 2xh + h^2$

• Step 3: Divide by $h$.
  $\dfrac{2xh + h^2}{h} = 2x + h$

Final Answer
The difference quotient for $f(x) = x^2$ is $(2x + h)$

Question: 2

Given $f(x) = 3x + 4$, calculate the difference quotient.

Solution:

• Step 1: Find $f(x + h)$.
  $f(x + h) = 3(x + h) 4 = 3x + 3h + 4$

• Step 2: Subtract $f(x)$ from $f(x + h)$.
  $(3x + 3h + 4) - (3x + 4) = 3h$

• Step 3: Divide by $h.$ $\dfrac{3h}{h} = 3$

Final Answer
The difference quotient for $f(x) = 3x + 4$ is $3$

Question: 3

Let $f(x) = \frac{1}{x}$. Find the difference quotient for this function and simplify the expression.

Solution:

Step 1: Write the difference quotient formula: $\dfrac{f(x + h) - f(x)}{h}$
We must compute $f(x + h)$ and $f(x)$, then substitute them into the formula.

Step 2: Find $f(x + h)$.
Given $f(x) = \dfrac{1}{x}$, we substitute $x h$ for $x$ in the function: $f(x + h) = \dfrac{1}{x + h}$

Step 3: Substitute $f(x + h)$ and $f(x)$ into the difference quotient formula.
Using $f(x + h) = \dfrac{1}{x + h}$ and $f(x) = \dfrac{1}{x}$, the difference quotient becomes: $\dfrac{\frac{1}{x+h} - \frac{1}{x}}{h}$

Step 4: Simplify the numerator.
To simplify $\dfrac{1}{x + h} - \dfrac{1}{x}$, we need a common denominator. The common denominator between $x$ and $x + h$ is $x(x + h)$. So, rewrite the expression: $\dfrac{1}{x + h} - \dfrac{1}{x} = \dfrac{x - (x + h)}{x(x + h)} = \dfrac{x - x - h}{x(x + h)} = \dfrac{-h}{x(x + h)}$

Step 5: Substitute this simplified numerator back into the difference quotient: $\dfrac{\frac{-h}{x(x + h)}}{h}$

Step 6: Simplify the entire expression.
Now, divide the numerator by $h$. This is the same as multiplying by $\dfrac{1}{h}$: $\dfrac{-h}{h \cdot x(x + h)} = \dfrac{-1}{x(x + h)}$

Final Answer:
The simplified difference quotient for $f(x) = \dfrac{1}{x}$ is: $\dfrac{-1}{x(x + h)}$

Question: 4

Let $f(x) = 3x^2 + 2x$. Find the difference quotient for this function and simplify the expression.

Solution:

Step 1: Write the difference quotient formula: $\dfrac{f(x + h) - f(x)}{h}$

We need to calculate $f(x + h)$, subtract $f(x)$, and then divide by $h$.

Step 2: Find $f(x + h)$.

Given $f(x) = 3x^2 + 2x$, we substitute $x h$ for $x$ in the function: $f(x + h) = 3(x + h)^2 + 2(x + h)$

Now expand both terms: $f(x + h) = 3(x^2 + 2xh + h^2) + 2(x + h) = 3x^2 + 6xh + 3h^2 + 2x + 2h$

Step 3: Substitute $f(x + h)$ and $f(x)$ into the difference quotient formula.
We already know that $f(x) = 3x^2 + 2x$, so substitute both $f(x + h)$ and $f(x)$ into the difference quotient: $\dfrac{(3x^2 + 6xh + 3h^2 + 2x + 2h) - (3x^2 + 2x)}{h}$

Step 4: Simplify the numerator.
Simplify the terms inside the parentheses: $(3x^2 + 6xh + 3h^2 + 2x + 2h) - (3x^2 + 2x) = 6xh + 3h^2 + 2h$

So the difference quotient becomes: $\dfrac{6xh + 3h^2 + 2h}{h}$

Step 5: Simplify the entire expression.
Now, divide every term in the numerator by $h$: $\dfrac{6xh}{h} + \dfrac{3h^2}{h} + \dfrac{2h}{h} = 6x + 3h + 2$

Final Answer:
The simplified difference quotient for $f(x) = 3x^2 + 2x$ is: $6x + 3h + 2$

8. Practice Questions on Difference Quotient:

Now that we've walked through some examples, it’s time to practice:

Q:1. For $f(x) = x^3$, find the difference quotient.

Q:2. Find the difference quotient for $f(x) = \sin(x)$.

Q:3. Calculate the difference quotient for $f(x) = 5x^2 - 2x + 1$.

These practice problems will help reinforce the method and understanding of the difference quotient.

9. FAQs on Difference Quotient:

What is the difference quotient in simple terms?

The difference quotient is a formula used to calculate a function's average rate of change between two points. It helps measure how a function’s output changes as the input changes.

How is the difference quotient formula written?

The difference quotient formula is written as $\dfrac{f(x+h)-f(x)}{h}$, where $f(x)$ is the function and $h$ represents the distance between two points on the x-axis.

Why is the difference quotient important in calculus?

The difference quotient is essential because it forms the basis for understanding derivatives. As the value of $h$ approaches zero, the difference quotient leads to the derivative, which measures the instantaneous rate of change.

Can the difference quotient be used for all types of functions?

Yes, the difference quotient can be applied to a wide range of functions, including polynomial, trigonometric, logarithmic, and exponential functions.

What does the difference quotient represent graphically?

Graphically, the difference quotient represents the slope of the second line connecting two points on the curve of a function. As $h$ approaches zero, this slope becomes the tangent line's slope.

What happens if $h = 0$ in the difference quotient?

If $h = 0$, the difference quotient becomes undefined because division by zero is not allowed in mathematics. However, we derive the derivative by taking the limit as $h$ approaches zero.

How is the difference quotient related to velocity in physics?

In physics, the difference quotient can be used to calculate the average velocity of an object over a time interval. It compares the change in position (displacement) to the change in time, similar to how it calculates rates of change in functions.

10. Real-life Application of Difference Quotient:

While the difference quotient might seem purely academic, it has real-world applications.

• Measuring Average Velocity: In physics, the difference quotient is used to calculate the average velocity of an object over a time interval. By comparing the change in position (displacement) to the change in time, we determine how fast an object moves between two points.

• Analyzing Stock Market Trends: Economists and financial analysts use the difference quotient to calculate the average rate of change in stock prices over time. This helps determine market trends, like whether a stock increases or decreases in value.

• Predicting Population Growth: In biology and demographics, the difference quotient helps calculate the average rate of population change over a certain period. Comparing population data over intervals offers insights into growth or decline trends.

• Assessing Speed in Manufacturing: In industrial settings, the difference quotient can be used to analyze the production rate by comparing the output of goods over time, helping optimize processes and identify inefficiencies.

11. Conclusion:

In conclusion, the difference quotient is a fundamental concept that introduces us to change in mathematics. Whether calculating slopes, predicting movement, or diving into the deeper waters of calculus, mastering the difference quotient opens doors to many mathematical applications. It is not just a stepping stone but a foundational building block in understanding the calculus of functions.

If you have any suggestions regarding the improvement of the content of this page, please write to me at My Official Email Address: [email protected]

$\fcolorbox{black}{lightpink}{\color{blue}{Get Assignment Help}}$
Are you Stuck on homework, assignments, projects, quizzes, labs, midterms, or exams?
To get connected to our tutors in real time. Sign up and get registered with us.

$\color{red} \bold{Related \space Pages:}$
Solving Algebraic Equations Calculator
Algebra Cheat Sheet
Pre Algebra Operation Calculators
Quadratic Equation Calculator
Greatest Common Divisior (GCD) Calculator