Beta Distribution Calculator

Get Homework Help

Neetesh Kumar | January 08, 2025 $\space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space$ Share this Page on:

When working with probabilities and proportions, the Beta Distribution is one of the most versatile tools available. The Beta Distribution Calculator for a Table makes it easier than ever to compute probabilities, mean, variance, and standard deviation for complex datasets. Whether you’re a statistician, researcher, or analyst, this calculator streamlines the process and delivers accurate results.

1. Introduction to the Beta Distribution Calculator

The Beta Distribution is a continuous probability distribution defined within the range $[0, 1]$. It’s widely used to model probabilities and random variables constrained to this interval, such as success rates, proportions, and probabilities in Bayesian statistics.

Our Beta Distribution Calculator is tailored for tabular data, offering a seamless way to compute key statistics like mean, variance, standard deviation, and more. Whether analyzing survey results, process reliability, or marketing probabilities, this tool is indispensable.

2. What is the Formulae used?

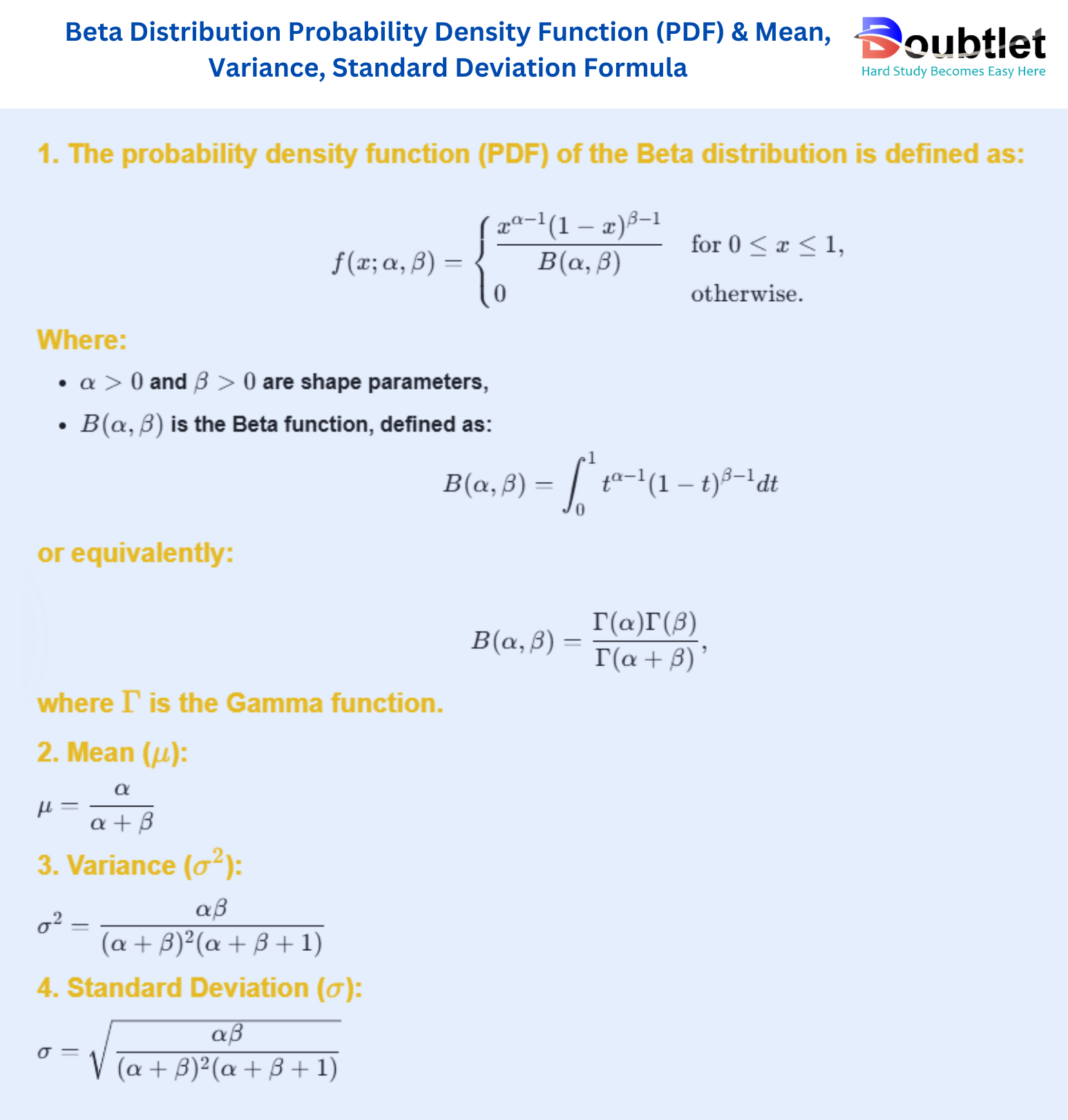
The Beta Distribution's key statistics are derived using the shape parameters $\alpha$ and $\beta$:

1. Probability Density Function (PDF):

   $f(x; \alpha, \beta) = \dfrac{x^{\alpha - 1}(1 - x)^{\beta - 1}}{B(\alpha, \beta)}$

   Where:

   • $x$: A value in $[0, 1]$.

   • $\alpha, \beta$: Shape parameters.

   • $B(\alpha, \beta)$: Beta function:

     $B(\alpha, \beta) = \displaystyle\int_{0}^{1} t^{\alpha - 1}(1 - t)^{\beta - 1} dt$

2. Mean ($\mu$):

   $\mu = \dfrac{\alpha}{\alpha + \beta}$

3. Variance ($\sigma^2$):

   $\sigma^2 = \dfrac{\alpha \beta}{(\alpha + \beta)^2 (\alpha + \beta + 1)}$

4. Standard Deviation ($\sigma$):

   $\sigma = \sqrt{\dfrac{\alpha \beta}{(\alpha + \beta)^2 (\alpha + \beta + 1)}}$

These formulas provide insights into the distribution's shape, spread, and behavior, all of which can be calculated effortlessly using our Beta Distribution Calculator.

3. How Do I Find the Beta Distribution?

To calculate Beta Distribution statistics manually:

1. Define Parameters: Identify $\alpha$ and $\beta$ based on your dataset.

2. Compute Mean: Use $\mu = \dfrac{\alpha}{\alpha + \beta}$.

3. Compute Variance: Use $\sigma^2 = \dfrac{\alpha \beta}{(\alpha + \beta)^2 (\alpha + \beta + 1)}$.

4. Find Standard Deviation: Take the square root of the variance.

5. Compute PDF: Substitute $x$, $\alpha$, and $\beta$ into the PDF formula.

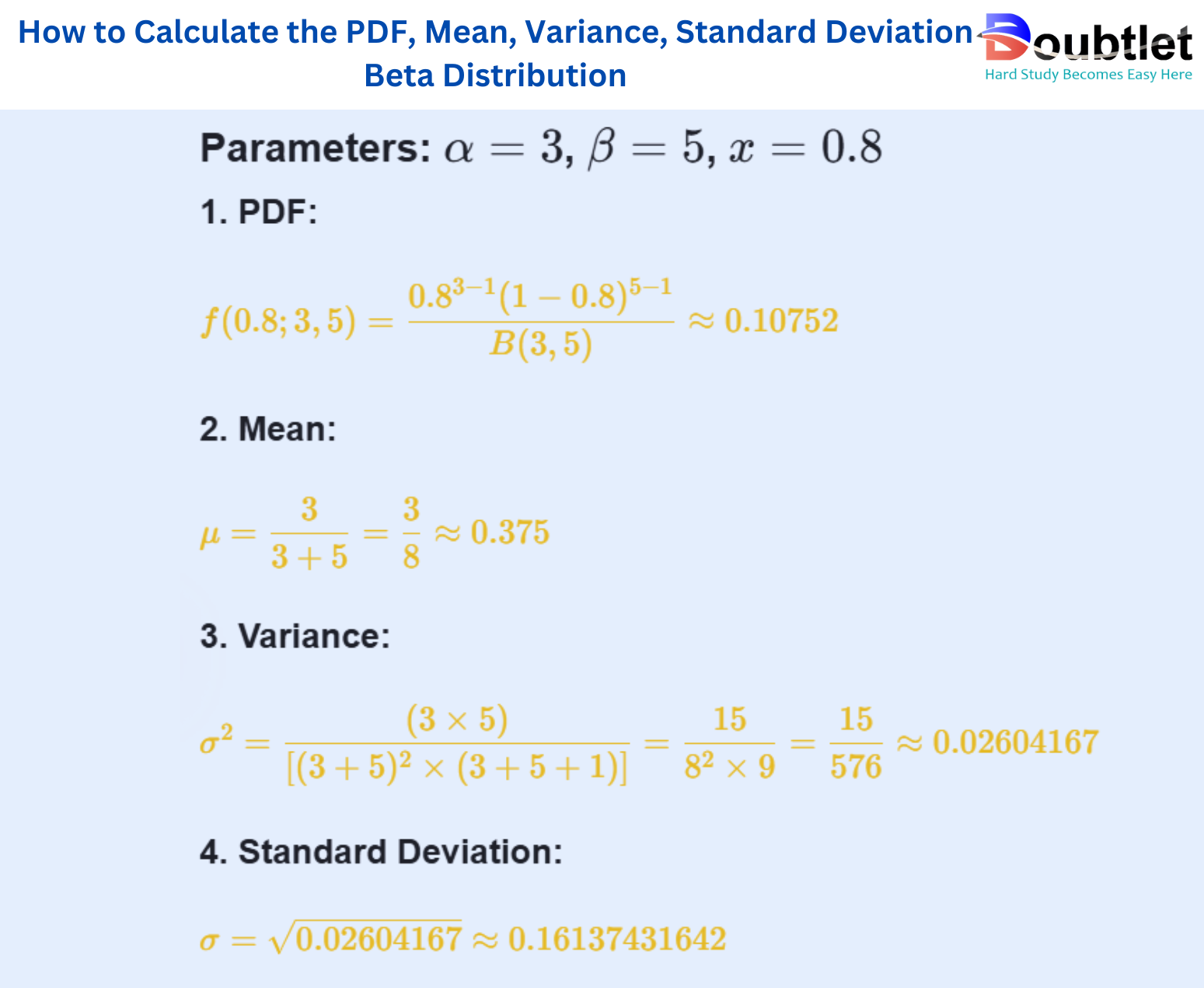
Example:
For $\alpha = 3$, $\beta = 5$:

• Mean:

  $\mu = \dfrac{3}{3 + 5} \approx 0.375$

• Variance:

  $\sigma^2 = \dfrac{(3 \times 5)}{[(3 + 5)^2 \times (3 + 5 + 1)]} \approx 0.02604166666$

• Standard Deviation:

  $\sigma = \sqrt{0.02604166666} \approx 0.16137430607$

With larger datasets, these calculations can become tedious, but our calculator handles them effortlessly.

4. Why Choose Our Beta Distribution Calculator?

$\bold{Easy \space \space to \space Use}$
Our calculator page provides a user-friendly interface that makes it accessible to both students and professionals. You can quickly input your square matrix and obtain the matrix of minors within a fraction of a second.

$\bold{Time \space Saving \space By \space automation}$
Our calculator saves you valuable time and effort. You no longer need to manually calculate each cofactor, making complex matrix operations more efficient.

$\bold{Accuracy \space and \space Precision}$
Our calculator ensures accurate results by performing calculations based on established mathematical formulas and algorithms. It eliminates the possibility of human error associated with manual calculations.

$\bold{Versatility}$
Our calculator can handle all input values like integers, fractions, or any real number.

$\bold{Complementary \space Resources}$
Alongside this calculator, our website offers additional calculators related to Pre-algebra, Algebra, Precalculus, Calculus, Coordinate geometry, Linear algebra, Chemistry, Physics, and various algebraic operations. These calculators can further enhance your understanding and proficiency.

5. A video based on how to Evaluate the Beta Distribution.

6. How to use this calculator?

Using the Beta Distribution Calculator is simple:

1. Input Parameters: Enter $\alpha$, $\beta$, and $x$.

2. Choose Output: Select the statistics you want to compute (PDF, CDF, mean, variance, etc.).

3. Click Calculate: Instantly get detailed results.

No complex formulas, no manual calculations just quick, accurate outputs.

7. Solved Examples on Beta Distribution

Example 1:

Find the Beta Distribution of the Dataset:

$\bigg[\alpha = \dfrac{2}{5}, \space \beta = 5.8, \space x = 0.3\bigg]$

Solution:

• Mean:

  $\mu = \dfrac{\frac{2}{5}}{\frac{2}{5} + 5.8} \approx 0.06451612903$

• Variance:

  $\sigma^2 = \dfrac{\big(\frac{2}{5}\times 5.8 \big)}{\big[\big(\frac{2}{5} + 5.8 \big)^2 \times \big(\frac{2}{5} + 5.8 + 1 \big)\big]} \approx 0.00838247196$

• Standard Deviation:

  $\sigma = \sqrt{0.00838247196} \approx 0.009155584066$

• PDF:

  $f\bigg(0.3; \dfrac{2}{5}, 5.8\bigg) = \dfrac{0.3^{\frac{2}{5}-1}(1 - 0.3)^{5.8-1}}{B(\frac{2}{5}, 5.8)} \approx 0.3315508$

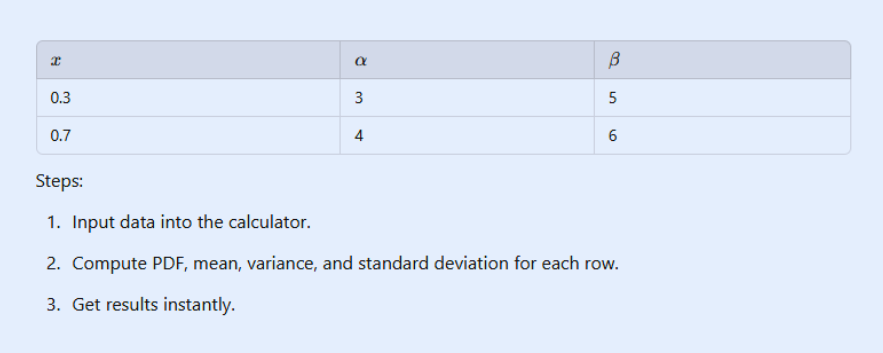
Example 2: Tabular Data:

8. Frequently Asked Questions (FAQs)

Q1. What is the Beta Distribution used for?

It’s used to model probabilities and proportions within the range $[0,1]$.

Q2. What do $\alpha$ and $\beta$ represent?

They are shape parameters that control the distribution's skewness and behavior.

Q3. Is this calculator free?

Yes, it’s completely free to use.

Q4. Does the tool handle large datasets?

Yes, it supports extensive tabular data.

Q5. Can it calculate CDF values?

Yes, the calculator computes both PDF and CDF values.

Q6. Is it mobile-compatible?

Yes, it works seamlessly on all devices.

Q7. Can I export results?

Yes, you can download results in multiple formats.

Q8. Does it show intermediate steps?

Yes, detailed steps are provided for better understanding.

9. What are the real-life applications?

The Beta Distribution has a wide range of applications, including:

• Finance: Analyze investment success probabilities.
• Project Management: Estimate task completion times in PERT analysis.
• Healthcare: Model probabilities in clinical trials.
• Marketing: Predict conversion rates for campaigns.
• Engineering: Assess reliability of systems and components.

Fictional Anecdote: Imagine Lisa, a project manager using the Beta Distribution Calculator to predict task completion probabilities. Armed with insights, she optimizes her schedule and improves project delivery by 15%.

10. Conclusion

The Beta Distribution Calculator is a vital tool for anyone working with probabilities, statistics, and data analysis. It simplifies complex calculations, saves time, and ensures accuracy, making it ideal for professionals, students, and researchers alike.

Ready to take control of your probability analysis? Try our Beta Distribution Calculator today and unlock new insights from your data!

If you have any suggestions regarding the improvement of the content of this page, please write to me at My Official Email Address: [email protected]

$\fcolorbox{black}{lightpink}{\color{blue}{Click here to Ask any Doubt}}$
Are you Stuck on homework, assignments, projects, quizzes, labs, midterms, or exams?
To get connected to our tutors in real time. Sign up and get registered with us.

$\color{red} \bold{Related \space Calculators}$
Pearson Correlation Coefficient Calculator
Coefficient of Variation Calculator
Poisson Distribution Calculator
P Value Calculator
Box and Whisker Plot Calculator
Z Score Calculator
Binomial Distribution Calculator