Matrix Column Space Calculator

Get Homework Help

Neetesh Kumar | February 3, 2025 (Updated) $\space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space \space$ Share this Page on:

The Column Space of a Matrix, also called the range of a matrix, is a fundamental concept in linear algebra. It represents the set of all possible linear combinations of the columns of a matrix, helping in solving systems of linear equations, transformations, and rank calculations.

Our Matrix Column Space Calculator simplifies this process by automating the computation, saving time, and ensuring accuracy. Whether you're a student, engineer, or researcher, this tool helps you effortlessly determine the column space, basis, and rank of a matrix.

1. Introduction to the Column Space of a Matrix

Have you ever pondered the significance of the "columns" within a matrix? The column space holds the key to unraveling the matrix's transformative power. In this blog, let's embark on a journey into the world of the column space, exploring its definition, applications, and how it reveals crucial insights about the matrix's structure and behavior.
$\bold{Definition}$
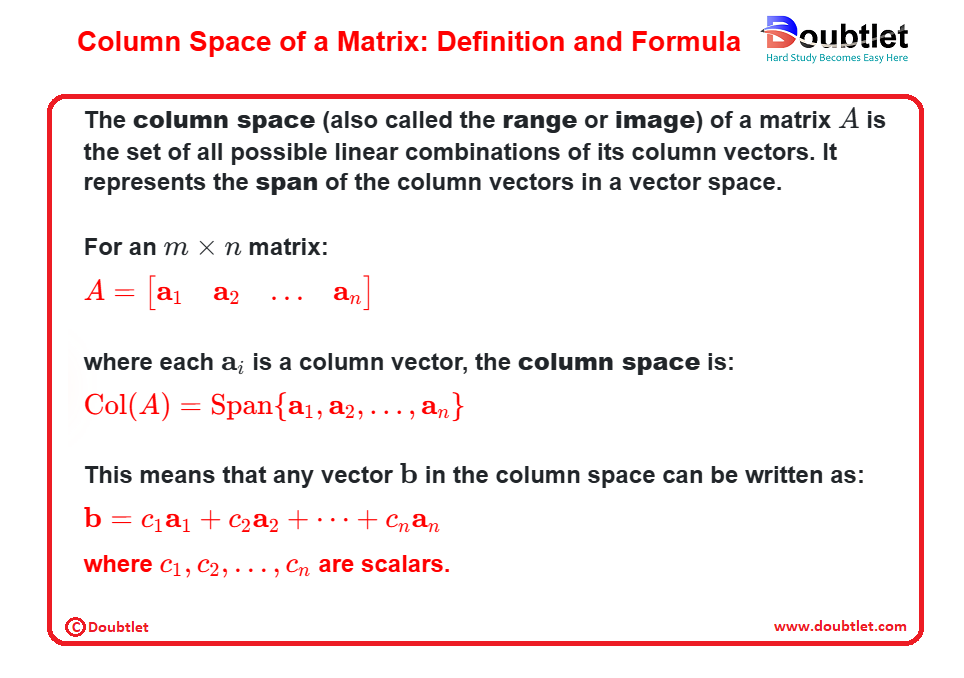
The column space of a matrix is the set of all possible linear combinations of its columns. In simpler terms, it represents the span of the columns, showcasing the diverse directions and magnitudes that can be formed using these columns. Understanding the column space is pivotal for grasping the matrix's transformations and relationships.

2. What is the Formulae used & conditions required?

$\bold{Formula \space used}$
The column space is determined by identifying the linearly independent columns of the matrix. $\bold{Conditions \space required}$
The condition for a basis of the column space is that the selected columns must be linearly independent. The formula involves finding the span of these columns.

3. How do I calculate the Column space of a matrix?

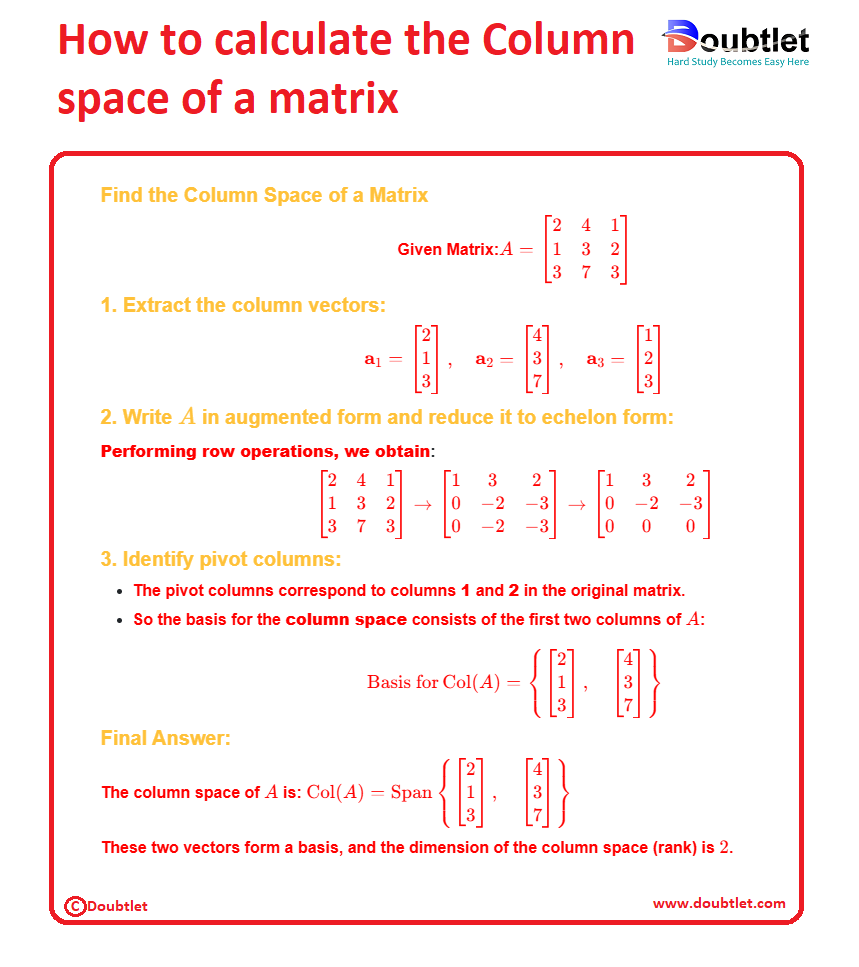
First, find the Reduced row echelon form of the given matrix.
Now, column space is a space spanned by the columns of the given matrix that corresponds to the pivot columns of the reduced matrix

Step 1: Convert the Matrix to RREF

The Reduced Row Echelon Form (RREF) helps determine the pivot columns, which form the basis of the column space.

Step 2: Identify Pivot Columns

Pivot columns in the RREF correspond to linearly independent columns in the original matrix.

Step 3: Form the Basis of the Column Space

The basis of Col(A) consists of the original matrix’s pivot columns.

Example Calculation:

Given:

$A = \begin{bmatrix} 1 & 2 & 3 \\ 2 & 4 & 6 \\ 3 & 6 & 9 \end{bmatrix}$

Step 1: Convert to RREF

$\begin{bmatrix} 1 & 2 & 3 \\ 0 & 0 & 0 \\ 0 & 0 & 0 \end{bmatrix}$

Step 2: Identify Pivot Columns

Only the first column contains a pivot (leading 1).

Step 3: Write the Basis of the Column Space

$\text{Basis} = \left\{ \begin{bmatrix} 1 \\ 2 \\ 3 \end{bmatrix} \right\}$

Thus, the column space is 1-dimensional, and its basis consists of the first column of the original matrix.

This process can be tedious, especially for large matrices our calculator automates it instantly!

4. Why choose the column space of a matrix calculator?

$\bold{Easy \space \space to \space Use}$
Our calculator page provides a user-friendly interface that makes it accessible to both students and professionals. You can quickly input your square matrix and obtain the matrix of minors within a fraction of a second.

$\bold{Time \space Saving \space By \space automation}$
Our calculator saves you valuable time and effort. You no longer need to manually calculate each cofactor, making complex matrix operations more efficient.

$\bold{Accuracy \space and \space Precision}$
Our calculator ensures accurate results by performing calculations based on established mathematical formulas and algorithms. It eliminates the possibility of human error associated with manual calculations.

$\bold{Versatility}$
Our calculator can handle all input values like integers, fractions, or any real number.

$\bold{Complementary \space Resources}$
Alongside this calculator, our website offers additional calculators related to Pre-algebra, Algebra, Precalculus, Calculus, Coordinate geometry, Linear algebra, Chemistry, Physics, and various algebraic operations. These calculators can further enhance your understanding and proficiency.

5. A video based on how to find the Column space of a Matrix.

6. How to use this calculator

This calculator will help you find a matrix's Column space.
In the given input boxes, you have to put the value of the given matrix.
After clicking on the Calculate button, a step-by-step solution will be displayed on the screen.
You can access, download, and share the solution.

7. Solved Examples on Matrix Column Space

$\bold{Question}$
Find the Column space of the given matrix = $\begin{bmatrix} 3 & 5 & 1 \\ 1 & 2 & 2 \\ 3 & 9 & 7 \end{bmatrix}$
$\bold{Solution}$
$\bold{Step \space 1:}$ Convert the above matrix to reduced row echelon form = $\begin{bmatrix} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{bmatrix}$.
$\bold{Step \space 2:}$ column space is a space spanned by the columns of the given matrix that corresponds to the pivot columns of the reduced matrix = {$\begin{bmatrix} 3 \\ 1 \\ 3 \end{bmatrix}$, $\begin{bmatrix} 5 \\ 2 \\ 9 \end{bmatrix}$, $\begin{bmatrix} 1 \\ 2 \\ 7 \end{bmatrix}$}

8. Frequently Asked Questions (FAQs)

Why is the column space of a matrix important?

The column space provides insights into the diverse directions that the columns of a matrix can span, offering a key to understanding its linear transformations and relationships.

Can the column space be larger than the number of columns in the matrix?

No, the column space cannot be larger than the number of columns. It is, at most, equal to the number of columns in the matrix.

What if the columns are not linearly independent?

If the columns are not linearly independent, the column space may not cover the entire space, and adjustments may be needed to ensure a basis for the column space.

Does changing the order of the columns affect the column space?

No, changing the order of the columns does not affect the column space. The column space is determined by the linear independence of the columns, not their order.

Can the column space be a subspace of a larger space?

Yes, the column space is a subspace of the space defined by the matrix. It represents a specific aspect of the entire space.

9. What are the real-life applications?

Understanding the column space is vital in fields like computer science, where matrices represent data structures and transformations. The column space helps extract meaningful information about the relationships between data points.

The Column Space Calculator is widely used in:

• Engineering: Signal processing, control systems.
• Computer Science: Machine learning, 3D graphics.
• Economics: Statistical modeling, financial forecasting.
• Physics: Quantum mechanics, relativity.
• Data Science: Dimensionality reduction techniques (PCA).

10. Conclusion

As we conclude our exploration into the column space of a matrix, remember that it serves as a gateway into the directional possibilities embedded in the columns. Embrace the simplicity and significance of the column space and witness how it unravels essential insights about the matrix's structure and transformations, influencing applications in diverse fields.

If you have any suggestions regarding the improvement of the content of this page, please write to me at My Official Email Address: [email protected]

$\fcolorbox{black}{lightpink}{\color{blue}{Click here to Ask any Doubt}}$
Are you Stuck on homework, assignments, projects, quizzes, labs, midterms, or exams?
To get connected to our tutors in real time. Sign up and get registered with us.

$\color{red} \bold{Related \space Calculators}$

$\bold{Rank \space of \space a \space matrix}$
$\bold{Gram \space Schmidt \space Calculator}$
$\bold{Solving \space a \space system \space of \space linear \space equation}$
$\bold{Reduced \space row \space echelon \space form \space of \space a \space matrix}$
$\bold{Linear \space Independence \space of \space vectors}$
$\bold{Null \space space \space or \space Kernel \space Or \space Nullity}$
$\bold{Row \space space \space of \space a \space matrix}$