Find a parametric representation for the lower half of the ellipsoid $7x^2 + 2y^2 + z^2 = 1$ . (Enter your answer as a comma-separated list of equations. Let $x$ , $y$ , and $z$ be in terms of $u$ and/or $v$ .)

Question :

Find a parametric representation for the lower half of the ellipsoid $7x^2 + 2y^2 + z^2 = 1$ . (enter your answer as a comma-separated list of equations. let $x$ , $y$ , and $z$ be in terms of $u$ and/or $v$ .)

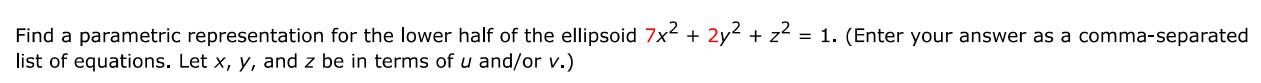
Find a parametric representation for the lower half of the ellipsoid $7x^2 + 2y^ | Doubtlet.com

Solution:

Neetesh Kumar | November 14, 2024

Calculus Homework Help

This is the solution to Math 1D
Assignment: 16.6 Question Number 13
Contact me if you need help with Homework, Assignments, Tutoring Sessions, or Exams for STEM subjects.
You can see our Testimonials or Vouches from here of the previous works I have done.

Get Homework Help

Step-by-step solution:

The equation of the ellipsoid is: $7x^2 + 2y^2 + z^2 = 1$

This is the equation of an ellipsoid with different coefficients for $x$ , $y$ , and $z$ , indicating different scaling along each axis. To find a parametric representation for the lower half of the ellipsoid, we can express $x$ , $y$ , and $z$ in terms of two parameters, $u$ and $v$ .

Step 1: Set up the Parametric Form

For an ellipsoid of this form, we can use spherical coordinates:

Let $x = \sqrt{\frac{1}{7}} \sin(u) \cos(v)$
Let $y = \sqrt{\frac{1}{2}} \sin(u) \sin(v)$
Let $z = \cos(u)$

The parameter $u$ will vary from $0$ to $\pi$ to cover the entire ellipsoid. However, since we want only the lower half, we restrict $u$ to $\frac{\pi}{2} \leq u \leq \pi$ , so that $z$ will be non-positive (i.e., $z \leq 0$ ).

Step 2: Final Parametric Equations

With this setup, the parametric equations for the lower half of the ellipsoid are: $x = \sqrt{\frac{1}{7}} \sin(u) \cos(v)$ $y = \sqrt{\frac{1}{2}} \sin(u) \sin(v)$ $z = -\cos(u)$

where:

$\frac{\pi}{2} \leq u \leq \pi$
$0 \leq v < 2\pi$

Answer:

The parametric representation for the lower half of the ellipsoid is: $x = \sqrt{\frac{1}{7}} \sin(u) \cos(v), \quad y = \sqrt{\frac{1}{2}} \sin(u) \sin(v), \quad z = -\cos(u)$