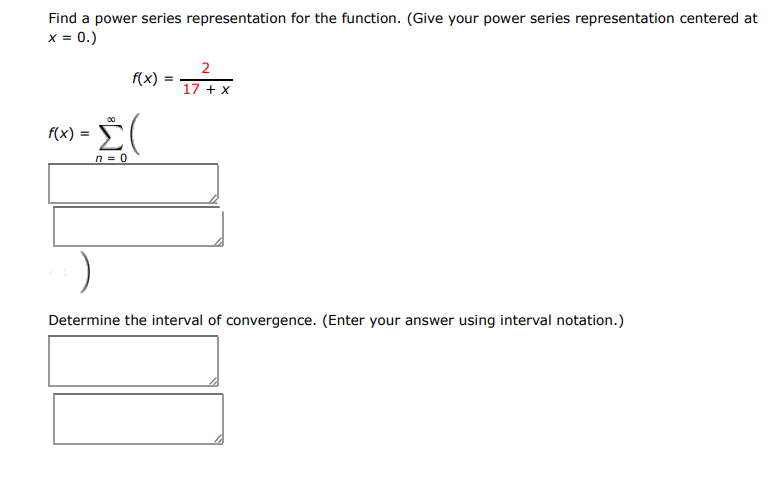
Find a power series representation for the function. (Give your power series representation centered at $x = 0$.) $f(x) = \frac{2}{17 + x}$

Determine the interval of convergence. (Enter your answer using interval notation.)

Neetesh Kumar | December 10, 2024

Calculus Homework Help

This is the solution to Math 132
Assignment: 11.9 Question Number 3
Contact me if you need help with Homework, Assignments, Tutoring Sessions, or Exams for STEM subjects.
You can see our Testimonials or Vouches from here of the previous works I have done.

Get Homework Help

---

Step-by-step solution:

Step 1: Rewrite the function

The given function is: $f(x) = \frac{2}{17 + x}.$

Factor $17$ from the denominator: $f(x) = \frac{2}{17(1 + \frac{x}{17})}.$

This simplifies to: $f(x) = \frac{2}{17} \cdot \frac{1}{1 + \frac{x}{17}}.$

Step 2: Use the geometric series formula

We know the geometric series formula: $\frac{1}{1 - r} = \displaystyle\sum_{n=0}^{\infty} r^n, \quad |r| < 1.$

Substitute $r = -\frac{x}{17}$ into the formula: $\frac{1}{1 + \frac{x}{17}} = \displaystyle\sum_{n=0}^{\infty} \left(-\frac{x}{17}\right)^n.$

Multiply by $\frac{2}{17}$: $f(x) = \frac{2}{17} \cdot \displaystyle\sum_{n=0}^{\infty} \left(-\frac{x}{17}\right)^n.$

Simplify: $f(x) = \displaystyle\sum_{n=0}^{\infty} \frac{2(-1)^n x^n}{17^{n+1}}.$

Thus, the power series representation is: $\boxed{f(x) = \displaystyle\sum_{n=0}^{\infty} \frac{2(-1)^n x^n}{17^{n+1}}}.$

Step 3: Determine the interval of convergence

The geometric series converges when: $\left| -\frac{x}{17} \right| < 1.$

Simplify: $\frac{|x|}{17} < 1.$

Multiply through by $17$: $|x| < 17.$

Thus, the interval of convergence is: $\boxed{(-17, 17)}.$

Final Answer:

1. Power series representation: $\boxed{f(x) = \displaystyle\sum_{n=0}^{\infty} \frac{2(-1)^n x^n}{17^{n+1}}}$

   
   

2. Interval of convergence: $\boxed{(-17, 17)}$

Please comment below if you find any error in this solution.
If this solution helps, then please share this with your friends.
Please subscribe to my Youtube channel for video solutions to similar questions.
Keep Smiling :-)