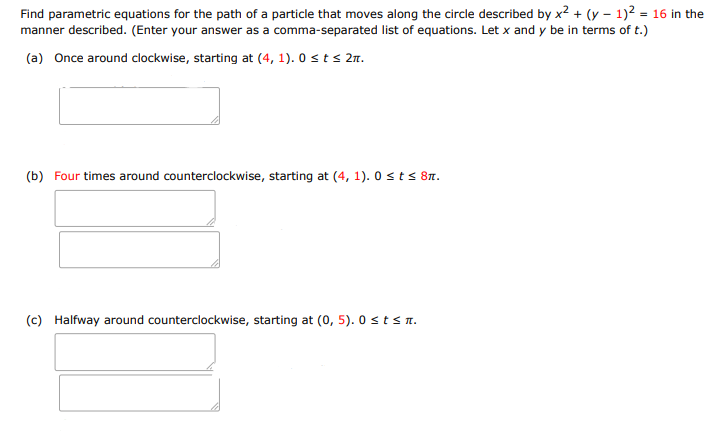
Find parametric equations for the path of a particle that moves along the circle described by $x^2 + (y - 1)^2 = 16$ in the manner described. (Enter your answer as a comma-separated list of equations. Let $x$ and $y$ be in terms of $t$.)

(a) Once around clockwise, starting at $(4, 1)$. $0 \leq t \leq 2\pi$.
(b) Four times around counterclockwise, starting at $(4, 1)$. $0 \leq t \leq 8\pi$.
(c) Halfway around counterclockwise, starting at $(0, 5)$. $0 \leq t \leq \pi$.

Neetesh Kumar | January 3, 2025

Calculus Homework Help

This is the solution to Math 1c
Assignment: 10.1 Question Number 9
Contact me if you need help with Homework, Assignments, Tutoring Sessions, or Exams for STEM subjects.
You can see our Testimonials or Vouches from here of the previous works I have done.

Get Homework Help

---

Step-by-step solution:

Understanding the Circle and Its Parametrization

The given circle is described by the equation:

$x^2 + (y - 1)^2 = 16$

This is the equation of a circle centered at $(0, 1)$ with a radius of $4$.

The general parametric equations for a circle are:

• $x = r\cos(t) + h$
• $y = r\sin(t) + k$

where $(h, k)$ is the center of the circle, and $r$ is the radius.

In this case, $h = 0$, $k = 1$, and $r = 4$. The parametric equations become:

• $x = 4\cos(t)$
• $y = 4\sin(t) + 1$

The direction (clockwise or counterclockwise) is determined by how $t$ progresses.

Part (a) Once Around Clockwise

For clockwise motion, the angle decreases with $t$.

Thus, we use $-t$ in the cosine and sine functions.

The parametric equations are:

• $x = 4\cos(-t)$
• $y = 4\sin(-t) + 1$

Using trigonometric identities ($\cos(-t) = \cos(t)$ and $\sin(-t) = -\sin(t)$):

• $x = 4\cos(t)$
• $y = -4\sin(t) + 1$

Answer for (a):

$x = 4\cos(t), y = -4\sin(t) + 1$ for $0 \leq t \leq 2\pi$.

Part (b) Four Times Around Counterclockwise

For counterclockwise motion, we use the regular parametric equations.

Since the particle goes around four times, the range of $t$ becomes $0 \leq t \leq 8\pi$.

The parametric equations are:

• $x = 4\cos(t)$
• $y = 4\sin(t) + 1$

Answer for (b):

$x = 4\cos(t), y = 4\sin(t) + 1$ for $0 \leq t \leq 8\pi$.

Part (c) Halfway Around Counterclockwise

Starting at $(0, 5)$ corresponds to the topmost point of the circle, which occurs when $t = \pi/2$.

From there, the particle moves counterclockwise halfway around the circle.

The range of $t$ is adjusted so that $t = 0$ corresponds to $(0, 5)$.

To achieve this, we shift the parameter $t$ by $\pi/2$:

• $x = 4\cos(t - \pi/2)$
• $y = 4\sin(t - \pi/2) + 1$

Using trigonometric identities ($\cos(t - \pi/2) = \sin(t)$ and $\sin(t - \pi/2) = -\cos(t)$):

• $x = -4\sin(t)$
• $y = 4\cos(t) + 1$

Answer for (c):

$x = -4\sin(t), y = 4\cos(t) + 1$ for $0 \leq t \leq \pi$.

Final Answers

(a) $\boxed{x = 4\cos(t), y = -4\sin(t) + 1}$

(b) $\boxed{x = 4\cos(t), y = 4\sin(t) + 1}$

(c) $\boxed{x = -4\sin(t), y = 4\cos(t) + 1}$

Please comment below if you find any error in this solution.
If this solution helps, then please share this with your friends.
Please subscribe to my Youtube channel for video solutions to similar questions.
Keep Smiling :-)