Neetesh Kumar | November 16, 2024
Calculus Homework Help
This is the solution to Math 1D Assignment: 16.6 Question Number 23 Contact me if you need help with Homework, Assignments, Tutoring Sessions, or Exams for STEM subjects. Testimonials or Vouches from here of the previous works I have done.
Get Homework Help
Step-by-step solution:
To find the surface area of a parametric surface, we use the formula:
A = ∬ R ∥ r u × r v ∥ d A A = \iint_R \|\mathbf{r}_u \times \mathbf{r}_v\| \, dA A = ∬ R ∥ r u × r v ∥ d A
where:
r u = ∂ r ∂ u \mathbf{r}_u = \frac{\partial \mathbf{r}}{\partial u} r u = ∂ u ∂ r u u u r v = ∂ r ∂ v \mathbf{r}_v = \frac{\partial \mathbf{r}}{\partial v} r v = ∂ v ∂ r v v v R R R u v uv uv 0 ≤ u ≤ 1 0 \leq u \leq 1 0 ≤ u ≤ 1 0 ≤ v ≤ 5 π 0 \leq v \leq 5\pi 0 ≤ v ≤ 5 π
Step 1: Compute r u \mathbf{r}_u r u r v \mathbf{r}_v r v
The parametric equation is:
r ( u , v ) = ⟨ u cos ( v ) , u sin ( v ) , v ⟩ \mathbf{r}(u, v) = \langle u \cos(v), u \sin(v), v \rangle r ( u , v ) = ⟨ u cos ( v ) , u sin ( v ) , v ⟩
Compute r u \mathbf{r}_u r u
∂ ∂ u ( u cos ( v ) ) = cos ( v ) \frac{\partial}{\partial u}(u \cos(v)) = \cos(v) ∂ u ∂ ( u cos ( v )) = cos ( v ) ∂ ∂ u ( u sin ( v ) ) = sin ( v ) \frac{\partial}{\partial u}(u \sin(v)) = \sin(v) ∂ u ∂ ( u sin ( v )) = sin ( v ) ∂ ∂ u ( v ) = 0 \frac{\partial}{\partial u}(v) = 0 ∂ u ∂ ( v ) = 0
Thus:
r u = ⟨ cos ( v ) , sin ( v ) , 0 ⟩ \mathbf{r}_u = \langle \cos(v), \sin(v), 0 \rangle r u = ⟨ cos ( v ) , sin ( v ) , 0 ⟩
Compute r v \mathbf{r}_v r v
∂ ∂ v ( u cos ( v ) ) = − u sin ( v ) \frac{\partial}{\partial v}(u \cos(v)) = -u \sin(v) ∂ v ∂ ( u cos ( v )) = − u sin ( v ) ∂ ∂ v ( u sin ( v ) ) = u cos ( v ) \frac{\partial}{\partial v}(u \sin(v)) = u \cos(v) ∂ v ∂ ( u sin ( v )) = u cos ( v ) ∂ ∂ v ( v ) = 1 \frac{\partial}{\partial v}(v) = 1 ∂ v ∂ ( v ) = 1
Thus:
r v = ⟨ − u sin ( v ) , u cos ( v ) , 1 ⟩ \mathbf{r}_v = \langle -u \sin(v), u \cos(v), 1 \rangle r v = ⟨ − u sin ( v ) , u cos ( v ) , 1 ⟩
Step 2: Compute the Cross Product r u × r v \mathbf{r}_u \times \mathbf{r}_v r u × r v
The cross product r u × r v \mathbf{r}_u \times \mathbf{r}_v r u × r v r u × r v = ∣ i j k cos ( v ) sin ( v ) 0 − u sin ( v ) u cos ( v ) 1 ∣ \mathbf{r}_u \times \mathbf{r}_v = \begin{vmatrix}
\mathbf{i} & \mathbf{j} & \mathbf{k} \\
\cos(v) & \sin(v) & 0 \\
-u \sin(v) & u \cos(v) & 1
\end{vmatrix} r u × r v = i cos ( v ) − u sin ( v ) j sin ( v ) u cos ( v ) k 0 1
Expanding the determinant:
For i \mathbf{i} i i ∣ sin ( v ) 0 u cos ( v ) 1 ∣ = i [ sin ( v ) − 0 ] = i sin ( v ) \mathbf{i} \begin{vmatrix} \sin(v) & 0 \\ u \cos(v) & 1 \end{vmatrix} = \mathbf{i} \left[\sin(v) - 0\right] = \mathbf{i} \sin(v) i sin ( v ) u cos ( v ) 0 1 = i [ sin ( v ) − 0 ] = i sin ( v )
For j \mathbf{j} j − j ∣ cos ( v ) 0 − u sin ( v ) 1 ∣ = − j [ cos ( v ) ( 1 ) − ( 0 ) ( − u sin ( v ) ) ] = − j cos ( v ) -\mathbf{j} \begin{vmatrix} \cos(v) & 0 \\ -u \sin(v) & 1 \end{vmatrix} = -\mathbf{j} \left[\cos(v)(1) - (0)(-u \sin(v))\right] = -\mathbf{j} \cos(v) − j cos ( v ) − u sin ( v ) 0 1 = − j [ cos ( v ) ( 1 ) − ( 0 ) ( − u sin ( v )) ] = − j cos ( v )
For k \mathbf{k} k k ∣ cos ( v ) sin ( v ) − u sin ( v ) u cos ( v ) ∣ = k [ u cos 2 ( v ) + u sin 2 ( v ) ] = k u \mathbf{k} \begin{vmatrix} \cos(v) & \sin(v) \\ -u \sin(v) & u \cos(v) \end{vmatrix} = \mathbf{k} \left[u \cos^2(v) + u \sin^2(v)\right] = \mathbf{k} u k cos ( v ) − u sin ( v ) sin ( v ) u cos ( v ) = k [ u cos 2 ( v ) + u sin 2 ( v ) ] = k u
Thus:
r u × r v = ⟨ sin ( v ) , − cos ( v ) , u ⟩ \mathbf{r}_u \times \mathbf{r}_v = \langle \sin(v), -\cos(v), u \rangle r u × r v = ⟨ sin ( v ) , − cos ( v ) , u ⟩
Step 3: Compute the Magnitude of r u × r v \mathbf{r}_u \times \mathbf{r}_v r u × r v
The magnitude is:
∥ r u × r v ∥ = sin 2 ( v ) + ( − cos ( v ) ) 2 + u 2 \|\mathbf{r}_u \times \mathbf{r}_v\| = \sqrt{\sin^2(v) + (-\cos(v))^2 + u^2} ∥ r u × r v ∥ = sin 2 ( v ) + ( − cos ( v ) ) 2 + u 2
Simplify:
∥ r u × r v ∥ = sin 2 ( v ) + cos 2 ( v ) + u 2 = 1 + u 2 \|\mathbf{r}_u \times \mathbf{r}_v\| = \sqrt{\sin^2(v) + \cos^2(v) + u^2} = \sqrt{1 + u^2} ∥ r u × r v ∥ = sin 2 ( v ) + cos 2 ( v ) + u 2 = 1 + u 2
Step 4: Set Up the Double Integral
The surface area is:
A = ∬ R 1 + u 2 d A A = \iint_R \sqrt{1 + u^2} \, dA A = ∬ R 1 + u 2 d A
Substitute the bounds for u u u v v v A = ∫ 0 5 π ∫ 0 1 1 + u 2 d u d v A = \int_0^{5\pi} \int_0^1 \sqrt{1 + u^2} \, du \, dv A = ∫ 0 5 π ∫ 0 1 1 + u 2 d u d v
Step 5: Evaluate the Inner Integral (with respect to u u u
Let:
I = ∫ 0 1 1 + u 2 d u I = \int_0^1 \sqrt{1 + u^2} \, du I = ∫ 0 1 1 + u 2 d u
Use the substitution u = sinh ( t ) u = \sinh(t) u = sinh ( t ) d u = cosh ( t ) d t du = \cosh(t) \, dt d u = cosh ( t ) d t 1 + u 2 = cosh 2 ( t ) 1 + u^2 = \cosh^2(t) 1 + u 2 = cosh 2 ( t )
When u = 0 u = 0 u = 0 t = 0 t = 0 t = 0 u = 1 u = 1 u = 1 t = sinh − 1 ( 1 ) t = \sinh^{-1}(1) t = sinh − 1 ( 1 )
The integral becomes:
I = ∫ 0 sinh − 1 ( 1 ) cosh 2 ( t ) d t I = \int_0^{\sinh^{-1}(1)} \cosh^2(t) \, dt I = ∫ 0 s i n h − 1 ( 1 ) cosh 2 ( t ) d t
Use the identity cosh 2 ( t ) = 1 2 ( cosh ( 2 t ) + 1 ) \cosh^2(t) = \frac{1}{2}(\cosh(2t) + 1) cosh 2 ( t ) = 2 1 ( cosh ( 2 t ) + 1 ) I = 1 2 ∫ 0 sinh − 1 ( 1 ) ( cosh ( 2 t ) + 1 ) d t I = \frac{1}{2} \int_0^{\sinh^{-1}(1)} (\cosh(2t) + 1) \, dt I = 2 1 ∫ 0 s i n h − 1 ( 1 ) ( cosh ( 2 t ) + 1 ) d t
Separate the terms:
I = 1 2 ∫ 0 sinh − 1 ( 1 ) cosh ( 2 t ) d t + 1 2 ∫ 0 sinh − 1 ( 1 ) 1 d t I = \frac{1}{2} \int_0^{\sinh^{-1}(1)} \cosh(2t) \, dt + \frac{1}{2} \int_0^{\sinh^{-1}(1)} 1 \, dt I = 2 1 ∫ 0 s i n h − 1 ( 1 ) cosh ( 2 t ) d t + 2 1 ∫ 0 s i n h − 1 ( 1 ) 1 d t
For ∫ cosh ( 2 t ) d t \int \cosh(2t) \, dt ∫ cosh ( 2 t ) d t 1 2 sinh ( 2 t ) \frac{1}{2} \sinh(2t) 2 1 sinh ( 2 t ) ∫ 1 d t \int 1 \, dt ∫ 1 d t t t t
Evaluate:
I = 1 2 [ 1 2 sinh ( 2 t ) ] 0 sinh − 1 ( 1 ) + 1 2 [ t ] 0 sinh − 1 ( 1 ) I = \frac{1}{2} \left[\frac{1}{2} \sinh(2t) \right]_0^{\sinh^{-1}(1)} + \frac{1}{2} \left[t\right]_0^{\sinh^{-1}(1)} I = 2 1 [ 2 1 sinh ( 2 t ) ] 0 s i n h − 1 ( 1 ) + 2 1 [ t ] 0 s i n h − 1 ( 1 ) I = 1 4 sinh ( 2 sinh − 1 ( 1 ) ) + 1 2 sinh − 1 ( 1 ) I = \frac{1}{4} \sinh(2 \sinh^{-1}(1)) + \frac{1}{2} \sinh^{-1}(1) I = 4 1 sinh ( 2 sinh − 1 ( 1 )) + 2 1 sinh − 1 ( 1 )
Simplify using sinh ( 2 x ) = 2 sinh ( x ) cosh ( x ) \sinh(2x) = 2\sinh(x)\cosh(x) sinh ( 2 x ) = 2 sinh ( x ) cosh ( x ) sinh − 1 ( 1 ) = ln ( 1 + 2 ) \sinh^{-1}(1) = \ln(1 + \sqrt{2}) sinh − 1 ( 1 ) = ln ( 1 + 2 )
Step 6: Evaluate the Outer Integral (with respect to v v v
After evaluating I I I v v v ∫ 0 5 π d v = 5 π \int_0^{5\pi} dv = 5\pi ∫ 0 5 π d v = 5 π
Thus:
A = 5 π ⋅ I A = 5\pi \cdot I A = 5 π ⋅ I
Final Answer:
The surface area is:
A = 5 π [ 1 4 sinh ( 2 sinh − 1 ( 1 ) ) + 1 2 sinh − 1 ( 1 ) ] A = 5\pi \left[\frac{1}{4} \sinh(2 \sinh^{-1}(1)) + \frac{1}{2} \sinh^{-1}(1)\right] A = 5 π [ 4 1 sinh ( 2 sinh − 1 ( 1 )) + 2 1 sinh − 1 ( 1 ) ]
Please comment below if you find any error in this solution.
If this solution helps, then please share this with your friends.
Please subscribe to my
Youtube channel for video solutions to similar questions.
Keep Smiling :-)










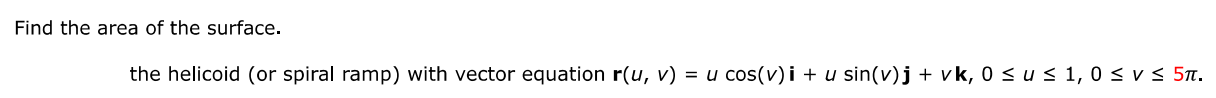




Leave a comment